We consider a complex population dynamics mathematical model involving foxes and rabbits as predators and prey. It is called the Lotka-Volterra model. Let r(t) be the number of rabbits and f(t) be the number of foxes. These are functions of time, and the time scale is rather long. The system of differential equations describing the rate of change of rabbits and foxes depends on four positive parameters , , 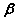, and 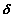. The mathematical model in two coupled, non-linear ODEs:

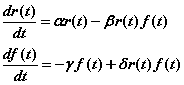
Where:
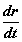is the rate of change of rabbits
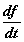is the rate of change of foxes
is the ‘birth-natural death’ rate for rabbits, and 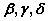are other constants that are part of the model.

The ODEs express the idea that the rate of change of the rabbits consists of two parts, one proportional to the number of rabbits (the first term) and the other, a negative contribution, is due to the interaction between rabbits and foxes (the second term). Note that because foxes eat rabbits, this second term is negative (the more foxes there are to eat rabbits, the less rabbits there will be). The rate of change of the foxes also consists of two parts. The first term is negative, and it is proportional to the number of foxes, because the more foxes there are, the harder it is for each fox to get food, so the population goes down. The second part is positive, and it says that if there are more rabbits, there is more food for foxes, so the population of foxes increases.

We first look for possible steady state solutions. By setting the derivatives to zero, we get

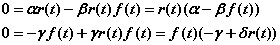
There are two solutions. The first has r(t)=f(t)=0. This is an uninteresting case. The second solution is

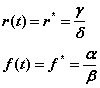
It can be shown that all the time dependent solutions are periodic with averages of rabbits and foxes equal to 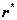and respectively.